How an Inline Skate Turns

c P. J. Baum
March 1999

Note Added 6-24-00-- See also Turning: Part II


 

1. The Turning Path Of An Inline Skate

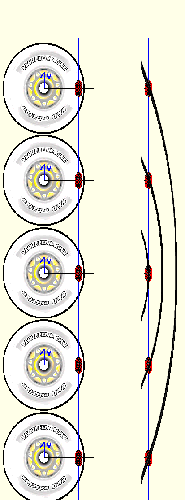
First I consider how a 5-wheel skate travels in "a circle" and then consider the basic turning process itself. The figure on the right shows five inline wheels leaning way left (exaggerated) with red-black contact patches where the wheels flatten against the floor. When the skate travels straight ahead along the blue line the contact patches are nicely in line. So if the skate were to travel a curved path either the contact patches must move out of line or the wheels must travel on different curves. It would seem to take a lot of energy to deform the wheels so that the contact patches move way out of line (unless you have very soft wheels) so I think the case of cornering for a speedskater follows the figure to the right.

The front wheel will be 1 and the rear wheel will be 5. So skating in a fairly sharp circle (10 foot diameter, 5 foot radius) the curves which pass through the middle wheel (3) and the curve which passes through 1 and 5 will be offset left-right by 0.3 inches or just over the size of the axle hole in the center of a bearing. So the curves are much straighter than they appear on my figure. If you slide there will be 5 curves instead of 3 as each wheel gets its own path. When you complete the turn the wheel paths will merge back to one if you move straight ahead. I am not aware of any real data on wheel paths but this seems most reasonable.

5 wheel path

A 2-wheel skate will have only one wheel-trajectory curve if you use the most efficient turn so it really can travel in a true circle. A 3-wheel skate and a 4-wheel skate will have only two curves. A 6-wheel skate will have three curves like the 5-wheel. We assumed here that all the wheels on the skate are the same size and shape.

 

2. How Does An Inline Wheel Turn?

Now that we have seen how the skate fits on a curved path it is time to explain how the wheels themselves can roll off a straight path. I am not concerned here with the hockey-slide type of turn or the type where all but one wheel is off the ground as they are inefficient or lack sufficient control for racing. To explain how the wheel turns we first need to know more about how they grip.

2a. Background on Wheel Grip

You can make these ideas on grip much clearer by demonstrating them for yourself. Locate a fairly new wheel which is soft. New means that it has a curved profile and a smooth surface. If the wheel is worn it will be difficult to observe the contact patches. If the wheel is hard it will be difficult to apply enough force with your hands to produce the contact patches. Put bearings in the wheel and slide a bolt through it and tighten a nut to hold the wheel. You should be able to hold onto the bolt and easily roll the wheel. Now find a piece of glass like an open window or glass table top where you have simultaneous access to both sides of the glass. Roll the wheel on one side of the glass and observe it from the other side. You should be able to see the contact patch where the wheel flattens against the glass. Get familiar with where the patch moves as you lean the wheel on edge. The wheel rolls ahead very easily. Now try to push or pull it in the direction of the bolt. If your wheels are like mine they have a pretty strong grip against sliding sideways. As you lay the wheel on edge (say 45 degrees) the sliding grip stays fairly strong. Now put the wheel straight up again and rotate the bolt in a circle parallel to the glass surface. It rotates fairly easily. Put the wheel on edge (45 degrees) and again the rotational grip is much weaker than the sliding grip.

Now get a pen and mark a small arrow on the bottom of the wheel (small enough to fit inside the contact patch if you were standing straight up on your skates). Point the arrow parallel to the bolt. Roll the wheel straight ahead and the arrow should maintain its direction as it rolls through the contact patch. This is how a wheel ought to behave. Right?

Ok, now mark an arrow farther on the side so it will fit into the contact patch when you place the wheel on edge at a 30-45 degree angle. Now roll the wheel straight ahead while it is on edge at the angle which will allow the arrow to contact the glass as it rolls. If you press the wheel against the glass lightly the arrow rotates as it passes through the contact patch. If you press hard the arrow stays at the angle it had when it entered the contact patch and then rotates quickly after it exits the contact patch. I conclude that if the wheel on edge is gripping rotationally, a torsional stress is built up on the surface of the wheel even as it rolls straight ahead!

This torsional stress starts as the arrow enters the contact patch and builds up to a maximum as it exits the contact patch. The level of torsional stress which can be built up can be controlled by the pressure you apply to the wheel. That is, the skater can determine when the rotational grip fails by selectively applying foot pressure. Because the sliding grip is so much stronger than the rotational grip the skater can probably modulate the rotational grip without destroying the sliding grip. Although it is possible that if you really push the turns, applying a lot of force, that when the rotational grip fails the torsional waves sent out from the point of failure may weaken the sliding grip locally. In this case both the rotational and sliding grip would be lost at the rear of the contact patch and would appear to move forward, toward the leading edge of the contact patch where the torsional stress is lowest.

2b. The Turning Process

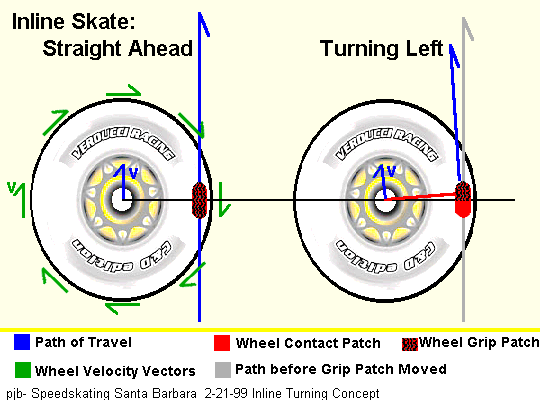
The left side of the figure below shows the wheel (and skate) moving straight ahead. The right side shows the proposed change during turning.

Left: The wheel is viewed from above looking down at the floor. The wheel is leaning way left (exaggerated). The wheel and skates move straight ahead along the blue line and the blue vector at the wheel axis shows the forward velocity there. The wheel makes contact with the floor at the red contact patch. The rotational grip patch is shown as black treadmarks. Note that the wheel as a whole moves forward at the speed v and every point on the periphery of the wheel (actually here a circle through the contact patch with center at the axle) moves with velocity v relative to the axle rotating around it. So long as the grip patch remains centered on the horizontal black line (connecting the axles on the left and right) the wheel and frame move straight ahead. Here the rotational component of velocity points directly behind the skater (down on the figure) but if you move the grip patch up or down just a little off the axle line there is a net horizontal velocity to the right or left.

The right side of the figure below shows the grip patch moved up a little or rotated forward through a small angle marked by the red line connecting the axle center with the grip patch center. Now the net velocity seen by the contact patch has a slight rightward rotational velocity which moves the wheel a little to the left.

 

Turning Method

3. Comments

It is this forward motion of the rotational grip (loss of the grip on the back portion of the contact patch where the torsional stress is largest) which allows the wheel to use the sideways motion of the wheel on edge to turn. Not surprisingly the turn is initiated by leaning to one side which builds up a torsional stress in the wheel's contact patch. When the rotational grip fails toward the trailing edge of the contact patch (through judicious choice of wheel compound, surface, speed ... or by judicious application of foot pressure) the grip moves forward and the skate turns.

There are a lot of complications which I will only point out briefly -- the contact patch cannot be formed or removed instantaneously. So when you move fast enough the contact patch length is not the same as you see at very low speeds. For car tires this effect is related to traction waves which distort the tire surface. For cars there is also a torsional wave but it is treated as separate from the traction wave because car tires do not lean on edge very far. For inline skate wheels on edge these waves are linked so at high speed the contact patch would be tied to torsional-traction-waves in an even more complex manner than car tire theory.

With the above understanding of turning it is not clear that the five-wheel skate is at any disadvantage over the 2-wheel skate for turning. The rolling-resistance and turning-resistance will be different for both but which is lowest needs more study. The two-wheel skate still has the advantage that larger diameter wheels can be used with their lower rolling-resistance. Would the losses be still lower for a hybrid skate with 2 large wheels in front-back and two or three regular wheels in between?

4. Acknowledgments

I appreciate helpful communications with Duncan Browne of the Inline Skating Newsgroup and from Dean Jackson of the Inline Racing Newsgroup. I found the series on The Physics of Racing--car racing-- by Brian Beckman to be helpful, especially Part 10: Grip Angle-- available on at least three servers on the internet.

 

Forward Stroking For Greater Efficiency

c. P.J. Baum, October 1999.


 

Introduction

Earlier I found that the efficiency of the sideways linear (straight-line, fixed-angle) stroke was only 50%. Here I examine the efficiency of a whole class of linear strokes of different angles finding efficiency geater than 50% for forward strokes and less than 50% for backward strokes. Still, the best stroke I have found is not a single linear stroke but involves a transition between two linear strokes -- one nearly sideways and one nearly forward.

Most skating is done in an attempt to accelerate the skater forward, but speedskating tries to accomplish this goal more powerfully and efficiently than other kinds of skating. Here I examine some of the strokes in the skater's toolbox for power and efficiency. The figure below shows how I would break stroking into three regions: backward, sideways, and forward.

 

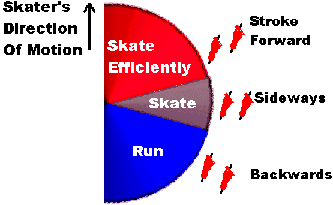
Run Skate Efficiency



Starting from the blue sector of the figure above, the skater basicly runs on his skates for the start. This backward stroke works well when you are at very low speed but propels the body forward at the expense of leaving the skate and leg behind in the dust. Moving the skate forward for the next stroke undoes a lot of the work you just did. Because of the inefficiency of the backward stroke the skater soon enters the gray "skate" region where the stroke is pretty much sideways. The skater spends a lot of time in this region where the skate now just keeps up with the skater's body. You push sideways and the skate is generous enough to convert half your work into forward motion retaining only the other half as its fee. The attempt to cajole the skate into giving up the other half of your energy is the subject of forward stroking which is the red region of the figure I labelled "skate efficiently". But before I look at the efficiency of forward stroking it should be pointed out that there are (at least) two types of forward strokes. I view them as passive and active.


 

Efficiency Of Active Forward Stroking

The figure below shows a skater's right foot and skate in relation to the force, F, the skater applies in an attempt to move forward. In the general case two angles are involved but to save space here I will discuss only a simpler case which has all the important elements but only one angle involved. For this case I have assumed that the angle between the skate and the forward direction equals the angle between the applied force and the skate. The left side of the figure shows the zero degree limit where the skate is pushed straight ahead. The right side shows the 45 degree case where you push directly sideways and move straight ahead. The arbitrary angle case is in the middle.
 

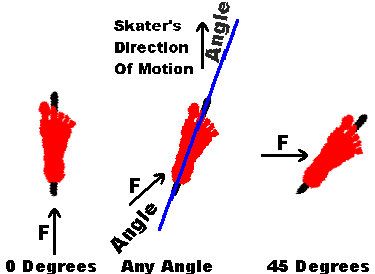
Stroke Angle


The solution is not difficult for this model and I have plotted the results below for energy (or power) efficiency. Pushing the skate straight ahead delivers all your energy to the skate (100% efficient) as you might expect. Pushing sideways delivers the 50% efficiency I found earlier. What is novel is that you can push for an unexpectedly large range of angles around the forward direction and still maintain an efficiency in the 90% range (the angle between the applied force and the forward direction is twice the angle plotted here so this range is even greater than it appears). Also, the efficiency of backward stroking (angles greater than 45 degrees) is less than 50%.

 

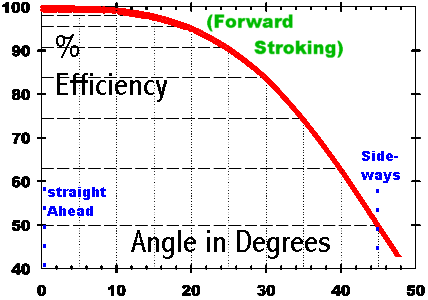
Efficiency vs Angle



The advantage of the active forward stroke is that you can skate up to small angles , not too far from straight ahead, and still react against the ground for power generation but not develop a lot of sideways energy to cut your efficiency. The disadvantage is exactly the opposite of the backward stroke. When stroking backwards your body gets ahead of your skates and while stroking forward you skates get ahead of your body. So start out conservatively or you may land on your backside. The forward stroke requires that your body be moved up to your skates for the next stroke. The situation is the same in the double push where the forward push is handled with body shifts, rotations, or thrusts depending on style. However, it seems a little harder to shift the body forward over the skates in the single-push (classic) style as the body is not so easily rotated in that case. Because of this limitation the amount of forward push is not too great and is best used briefly -- for example as the termination of a standard sideways push to develop additional forward thrust (see drawings here).

 

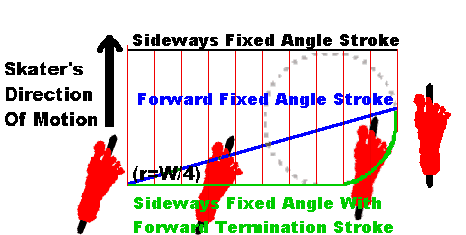
Sideways Forward Image


Since the efficiency of the forward stroke is greater than the sideways stroke it is tempting to try to use it exclusively. This turns out not to be advantageous because the shortest distance between two points is a straight line. That is, any linear stroke will have a short stroke path length so that although the efficiency may be high, the amount of energy generated along the short path is not maximum. The figure above shows a forward fixed-angle stroke and also a transition from a sideways to a forward stroke which ends at the same point. It seems fairly obvious that the path length of the linear stroke is shorter than the sideways-forward stroke so it will generate less energy. On the question of efficiency, the termination of the sideways stroke with the forward stroke rehabilitates the sideways stroke so that its overall efficiency will be comparable with or possibly exceed that of the single linear forward stroke.

Finally there are some conclusions to be drawn from the efficiency model used here. Recall these efficiencies: sideways 50%, forward 100%, midway between these ~90% (push 45 deg. off forward with skate 22.5 deg. from forward). So by pushing at 45 degrees from forward you gain 40% over sideways but at straight ahead you gain only an additional 10%. In other words you don't need to push anywhere near straight ahead to get a very high efficiency (90%). This allows an interesting possibility for stroke termination -- by terminating the stroke past straight ahead (around the corner and back toward the center line at a 45 degree angle) you can extend the stroke path length even further -generating even more energy- and suffer only a 10% drop in efficiency. This in fact seems to happen in the double push. I haven't worked out the overall result of this but it does have the advantage that the 10% "lost" energy due to inefficiency is used to move the skate back to the center line presumably resulting in a decreased interstroke time -- i.e. a faster stroke rate along with a possibly increased overall forward power due to the increased stroke path length.

"Exact" Nonlinear Stroke Model

c. P.J. Baum, December 1999.


11/25/99
 

1. Introduction

On an earlier page I had discussed coupling a linear sideways stroke with a forward turning stroke to increase the energy output of a skating stroke. Such a situation is shown below where the sideways skate angle and stroke position are shown as the right foot moves to the right. The analysis was an estimate only and the intent of this page is to quantitatively describe the stroke. The word "Exact" in the title is in quotes because when all is said the solution turns out to be close to the one we wanted but not quite the same. Nevertheless, the solution is highly instructive and points to the correct answer for several questions.

 

2. The Stroke Model

In the figure below the proposed stroke is shown with time now plotted across the bottom and not distance as before. The stroke is completed in time T and begins with a linear stroke (red feet) from time t=0 up to t=aT where a is some number between zero and one to be determined. After that time the stroke is completed with a nonlinear turning stroke (multicolored feet).

The direction of the force (F0) the skater applies is also shown. The force model comes from a forward-stroking model I used earlier where the problem is reduced to only one angle because the angle between the force and the skate is equal to the angle between the skate and the direction of motion (forward)-- see below.

 

The stroke starts out sideways and turns to straight ahead (Angle starts at 45 degrees and turns to 0 degrees). So The turning stroke is modelled as a sequence of linear strokes of different angle. No cornering properties of the skate or wheels is included but the force splitting is based on an absence of linear sliding. It turns out, that the linear stroke always loses (in this model anyway) and that the skate ends the stroke at highest velocity for a=0 (no linear portion at all). So the solution now becomes simpler with only a nonlinear portion. The skate angle as a function of time, t, is:

 

Angle(t) = [Pi/4]*[1-t/T]


where Pi/4 is 45 degrees expressed in radians. Now the model has the forward force varying as cos(Angle)^2 while the sideways force varies as sin(Angle)^2. So the terminal forward speed of the skate will be given by a time integral of cos(Angle)^2.

2a. Results: Stroke Efficiency With A "Simple Pull"

The exact solution to the integral equation for forward velocity is:

 

vf(t)=[F0/2M]*[t-{2T/Pi}*{cos(Pi/2*t/T)} +2T/Pi]

which has as its value at t=T
 

vf(T) = [F0*T/M]*[1/2 +1/Pi]=0.818*[F0*T/M]


So this model ended up with 81.8% of the maximum velocity available or 66.9% of the maximum energy available (vf ^2). This is a little disappointing but the reason for only a modest improvment over a linear stroke becomes clearer when we find that at time t=T that the sideways energy has not dropped to zero.

In fact, the force must be applied for an additional 8 degrees (turning back toward the skater's center line) before the sideways energy drops to zero. Then the forward velocity increases to 91.9% of the maximum available and the energy increases to 84.5% (even when the additional energy input from the longer stroke time is considered). If you look at the force arrows in the diagrams above only outward push forces were applied and it was not until an inward "simple pull" force was used (for the additional 8 degrees) that the sideways motion was really halted. We had to oversteer the skate to get it to fully turn the corner. So this model is for a skate/skater with very poor cornering characteristics.

 

2b. Efficiency Using A "Complex Pull"

2b1. How the "Complex Pull" Results from the "Cornering Force"

The model above is rather like a poor-handling car where the cornering can be improved by taking advantage of the tire's "Grip Angle" or "cornering force" [The Physics of Racing, Part 10: Grip Angle by Brian Beckman]. As Beckman explains, turning the steering wheel does not necessarily turn the car unless a real force (cornering force) is developed to pull the tire into the turn. The difference between the direction you steered and the direction the car moves is the "grip angle" and it will be near zero for a car which handles well. This cornering force is due to a differential grip across the tire's contact patch caused by the torsional stress on the tire and is independent of any linear (sideways) sliding or "slip". Unfortunately I have found no quantitative theory or model for the cornering force so I can only describe it qualitatively at this time. The figure below shows the turning concept I developed earlier for the inline skate wheel. The normal "push" force has caused the skate to move straight ahead along the blue line in the left panel and here the grip (tread pattern) is uniform throughgout the contact patch. As the skater executes the turn in the right panel the torsion increases in the contact patch causing the grip to move forward. This results in the wheel being "pulled" left away from the direction a linear "push" would move it.

 

Inline skate cornering has not progressed beyond the qualitative model so no quantitative results for efficiency can be obtained now. It may be noted however, that the pull from the cornering force develops a velocity component perpendicular to the skate which is absent in the linear push. This force first pulls the skate forward, assisting the push in propulsion, and then pulls the skate back to the skater's center line shortening the stroke time.

On ice or snow the cornering force is developed through the "carving turn" whereas on inlines the skater manipulates the wheel's contact patches to develop the cornering force. The skater pushes down with the heel to enlarge the rear contact patch and increase its torsion while rolling. The larger contact patch means the turn can be sharper if enough torsion is developed. In addition the outward heel push increases the rear wheel's contact patch torsion still more and the result is the cornering force which pulls you around the corner while the push helps the skate ahead.

 

3. Summary And Conclusions